
www.VirtualNerd.com
How Do You Graph a Quadratic Equation with No Solution?
Solve x2 - 4x + 5 = 0 by graphing.
Summary
- x=-b/(2a) is the formula for the axis of symmetry
- 22-4(2)+5=4-8+5=1
- Let's create a table to help us graph!
- That yellow dotted line is the axis of symmetry, and it cuts our parabola in half!
- The parabola doesn't cross the x-axis, which means there are no zeros for this polynomial
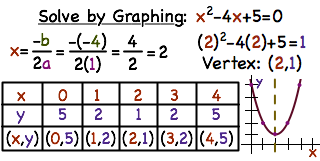
Notes
-

-

-

-

-
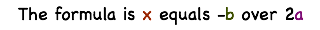
- This is the formula for the axis of symmetry
-

- Now we can plug these into the formula of the axis of symmetry:
- It's x=-b/(2a)
-

- -(-4)=4
- 2(1)=2
- 4/2=2
-

- The vertex is either the maximum or minimum value of the parabola
-

-

- Our axis of symmetry is x=2, so the vertex must have an x value of 2
-

- Since 2 is the x-value of our vertex, we can find the y-value by plugging 2 in for x in our original equation
- We get 22-4(2)+5 after pluggin in
- That reduces to 4-8+5, which is equal to 1
-

-

-

-

-

-

- We're plugging 0, 1, 2, 3, and 4 into the polynomial x2-4x+5
- Simplifying we get the points:
- (0,5)
- (1,2)
- (2,1)
- (3,2)
- (4,5)
-

-

-

-

-

- Remember, our axis of symmetry was the vertical line x=2
-

-

- The zeros of a function are where the graph crosses the x-axis
- They are the points where y=0
- These points will be the solutions to our equation
-

- Zeros are the points where y=0
-

-

-

- Since we can never make the left hand side of our equation equal 0, there are no solutions to this equation
-
