
www.VirtualNerd.com
How Do You Find the a, b, and c Values of a Quadratic Function?
Identify the a, b, and c values of y = x2 + 12x + 32.
Summary
- Remember, the standard form of a quadratic looks like ax2+bx+c, where 'x' is a variable and 'a', 'b', and 'c' are constant coefficients
- Knowing 'a', 'b', and 'c' helps you solve quadratic equations!
- When a coefficient is missing in front of a variable, you know that it's just equal to 1 :)
- 'c' is the constant term in the quadratic equation because it's not attached to an 'x' variable
- An equation without an x2 is not a quadratic equation, it's linear
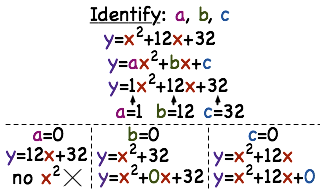
Notes
-

- Remember, the standard form of a quadratic looks like ax2+bx+c, where 'x' is a variable and 'a', 'b', and 'c' are constant coefficients
- ax2 is called the quadratic term, bx is the linear terms, and c is the constant term
-

- Knowing 'a', 'b', and 'c' helps you solve quadratic equations!
- The 'a' value is the coefficient in front of 'x2'
- In a quadratic equation, 'a' can't be zero! If it is zero, then you lose your quadratic term :)
-

- Knowing 'a', 'b', and 'c' helps you solve quadratic equations!
- The 'b' value is the coefficient in front of 'x'
-

- 'c' is the constant term, the term that doesn't contain x raised to some non-zero power
- Watch out for tricks! You could write c = c•x0, since x0=1!
-

- Let's look at what happens when 'a', 'b', and 'c' take on special values!
-

- To make y=12x+32 look like ax2+bx+c, you need to make a=0, b=12, c=32. But 'a' can't be zero in standard quadratic form, since 'a'=0 turns the equation into a linear equation!
- If you don't see an x2 term, you don't have a quadratic equation!
-

- In the case of y=x2+32, a=1, c=32, and there is no 'x' term. That means that 'b' just equals 0!
-

- In the case of y=x2+12x, a=1, b=12, and there is no constant term. That means that 'c' just equals 0!