
www.VirtualNerd.com
How Do You Solve a System of Equations Using the Elimination by Multiplication Method?
Find the solution to the following system of equations.
4x – 2y = 4 and 2x + y = 6
Summary
- We have 2 equations and 2 variables, so we've got a system of equations on our hands.
- Variables are unknown values, such as 'x' and 'y'.
- We can eliminate 'x' when adding the two equations together by multiplying 2x+y=6 by -2.
- After eliminating 'x', we are left with an equation that only has 'y'. This new equation can be solved in two-steps!
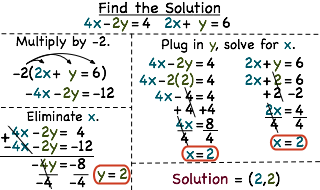
Notes
-

- When you've got multiple equations that share variables, you've got yourself a system of equations!
-

- Variables are unknown values, such as 'x' and 'y'.
- To make progress, you can try to combine the equations and eliminate one variable. In this system getting rid of x leaves an equation with only y, and solving an equation with 1 variable is easier than solving an equation with 2 variables!
- To get rid of x, we need to multiply 2x+y=6 by -2. Doing that will give us a -4x term, which will cancel with 4x when we add
-

- Variables are unknown values, such as 'x' and 'y'.
- To get rid of x, we need to multiply 2x+y=6 by -2. Doing that will give us a -4x term, which will cancel with 4x when we add
-

- Variables are unknown values, such as 'x' and 'y'.
- We find just one solution to this equation: x=2, y=2. We can write that as the ordered pair (2,2).
- Since there's only one solution to this system of equations, the system is called consistent and independent.