
www.VirtualNerd.com
How Do You Graph a System of Equations With No Solution?
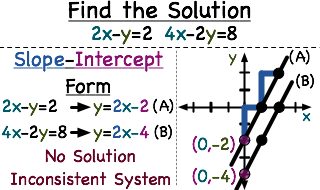
Find the solution(s) to the following system of equations: 2x – y = 2 and 4x – 2y = 8
Summary
- 'x' and 'y' are the variables in the given system of two equations
- To find the solution, we need to find the values of 'x' and 'y' that make both equations true
- Converting the given equations to slope-intercept form will help us graph the equations
- (A) stands for y=2x-2
- (B) stands for y=2x-4
- When we graph (A) and (B), we get two parallel lines!
- The solution to the given solution is the intersection of (A) and (B). Since parallel lines never cross, there is no solution!
- A system of equations with no solution is 'inconsistent'.
Notes
-

- Converting the given equations to slope-intercept form will help us graph the equations
- Slope-intercept form looks like y=mx+b, where m is the slope of the line, and b is the y-intercept of the line
- 'x' and 'y' are the variables in the given system of two equations
-

- We need to take 2x-y=2, and convert it to slope-intercept form
- (1): Use the subtraction property of equality to subtract 2x from both sides and isolate -y
- (2): Use the multiplication property of -1 and multiply both sides by -1 to convert -y to y
- If you'd like to see this done in more detail, check out the last link below!
-

- We need to take 4x-2y=8, and convert it to slope-intercept form
- (1): Use the subtraction property of equality to subtract 4x from both sides and isolate -2y
- (2): Use the multiplication property of -1 and multiply both sides by -1 to convert -2y to 2y. Then divide both sides by 2 to isolate y!
-

- The given equations are lines in the coordinate plane, and the solution to the given system is where the lines cross!
- The coordinate plane is the space that consists of the x and y-coordinate axes.
- The lines are in slope-intercept form, which lets you see the slope and intercept of each line without much trouble!
-

- The slope is positive 2, and that means that going up 2 units and over 1 unit will take us from one point to another
-

- Remember, the intersection of the graphed lines will give you the solution to the given system!
- Notice that the graphed lines are parallel though!
- Just looking at the slope-intercept form of the line tells you that these lines are parallel. See the last link below for more about that!