
www.VirtualNerd.com
How Do You Solve a System of Equations Using the Elimination by Addition Method?
Find the solution to the following system of equations: 2x–y=2 and 2x+y=6
Summary
- We're adding these equations together because the -y and +y will cancel out when added together
- We used the elimination method to find the value for x, it's 2
- We can plug x=2 into our original equations to find the value for y
- Our y-value is 2!
- Since there is only one solution to this system, we know it's consistent and independent
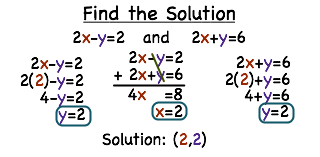
Notes
-

-

- We could use addition, subtraction, or multiplication when using the elimination method
-

-

-

-

-

- The first equation is 2x-y=2
- The second equation is 2x+y=6
-

-

- We've already done that for you in this example
-

- 2x and 2x are like terms, so we can combine them
- -y and y are also like terms, so we can combine them as well
- Constants like 6 and 2 are always like terms, so we can combine those also
-

- Since we added, the -y and +y canceled out
- Now dividing both sides by 2 we get x=2
-

-

- We're plugging x=2 into the equations 2x-y=2 and 2x+y=6
-

- The first equation was 2x-y=2
-

- Multiplying 2•2 gives us 4-y=2
- Then we can add y to both sides and subtract 2 from both sides to get y=2
-

- Let's check to make sure
-
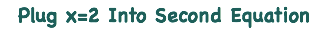
- The first equation was 2x+y=6
-

- Multiplying 2•2 gives us 4+y=6
- Subtracting 4 from both sides, we get y=2
-

- This means that y is definitely equal to 2
-

-

-
