
www.VirtualNerd.com
What's the Constant of Variation?
Why is it called a constant of variation?
Summary
- y=kx is the general form for writing a direct variation
- 'y' and 'x' are variables, while 'k' stands for a constant
- You can make a table to figure out the ordered pairs that satisfy the example y=2x
- Solve the direct variation equation for the constant of variation by dividing y values by x values
- xy=k is the general form for writing an inverse variation
- With inverse variation, you can find the constant of variation by multiplying x and y values, instead of dividing them
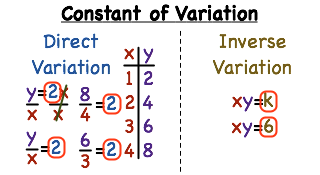
Notes
-

-

-

-

- 'y' and 'x' are variables, while 'k' stands for a constant
-

- 'k' stands for a constant, and is the constant of variation
- Notice that y=2x is in the form of y=kx
-

- Notice that y=2x is in the form of y=kx
- 'k' stands for a constant, and is the constant of variation
-

-

- Notice that y=2x is in the form of y=kx, where k is the constant of variation
-

-

- To build up the table, we just plug x-values into y=2x, and then write in the x- and y-values that we find!
-

- To build up the table, we just plug x-values into y=2x, and then write in the x- and y-values that we find!
- Notice we're just doubling x to find y : )
-

- Our example is y=2x, and the equation for direct variation is y=kx
- That makes our constant of variation k=2
-

- Notice that y=2x is in the form of y=kx, where k is the constant of variation
- When you just look at the equation, you can tell that the 2 is in the place of the 'k'. That's one way of figuring out the constant of variation:)
-

- We solved y=2x for 2 by dividing by x
- So if you have a table of values for a direct variation equation, dividing y by x will give you the constant of variation!
-

-

-

- Dividing y by x will get you the constant of variation in a direct variation problem!
-

-

-

- y=kx is the general form for writing a direct variation
- 'y' and 'x' are variables, while 'k' stands for a constant
- This means that if we have an ordered pair for our inverse variation, then we can multiply the x- and y-values together to get our constant of inverse variation
-

-

-

-

-

-
