
www.VirtualNerd.com
How Do You Find the Slope of a Ramp If You Know the Rise and Run?
Tony Hawk wants to design a ramp for one of his stunts. He finds that he can soar farthest if the ramp has a rise of 10 feet and a run of 20 feet. What is the slope of the ramp?
Summary
- Drawing a picture of Tony Hawk's ramp will help to visualize the problem better, so draw the ramp on a coordinate plane
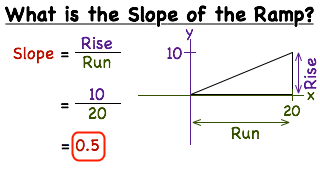
- 'Rise' is the change in y
- 'Run' is the change in x
- The ramp's rise is 10 feet
- The ramp's run is 20 feet
- We can find the slope of the ramp by dividing the rise, 10, by the run, 20
- Slope of the Ramp = 10/20 = 1/2 = 0.5
Notes
-

-

- Drawing a picture of Tony Hawk's ramp will help to visualize the problem better
-

- Drawing a picture of Tony Hawk's ramp will help to visualize the problem better
- The rise, or height, of the ramp is 10 feet above the ground
- The run, or length, of the ramp is 20 feet
-

- The rise, or height, of the ramp is 10 feet above the ground
- The run, or length, of the ramp is 20 feet
- 'Rise' is another word for the y-coordinate axis
- 'Run' is another word for the x-coordinate axis
-

- Drawing a picture of Tony Hawk's ramp will help to visualize the problem better
- The rise, or height, of the ramp is 10 feet above the ground
- The run, or length, of the ramp is 20 feet
- 'Rise' is another word for the y-coordinate axis
- 'Run' is another word for the x-coordinate axis
-

- 'Rise' is another word for the y-coordinate axis
-

- 'Run' is another word for the x-coordinate axis
-

- The rise, or height, of the ramp is 10 feet above the ground
- 'Rise' is another word for the y-coordinate axis
-

- The run, or length, of the ramp is 20 feet
- 'Run' is another word for the x-coordinate axis
-

- Drawing a picture of Tony Hawk's ramp will help to visualize the problem better
-

- We drew a picture of Tony Hawk's ramp to visualize the problem better
- Then we defined the rise and run of the ramp
- The final step is to figure out the slope of the ramp
-

-

- Slope is just the rise (change in y) over the run (change in x)
-

- Slope is just the rise (change in y) over the run (change in x)
- The rise, or height, of the ramp is 10 feet above the ground
- The run, or length, of the ramp is 20 feet
-

- Slope is just the rise (change in y) over the run (change in x)
- The rise, or height, of the ramp is 10 feet above the ground
- The run, or length, of the ramp is 20 feet
-

- Slope = Rise/Run
-

- Slope = Rise/Run
- Rise=10
- Run=20
-

- Slope = Rise/Run
- Rise=10
- Run=20
- Slope = 10/20 = 1/2 = 0.5
-

- Slope = 10/20 = 1/2 = 0.5
-

- Slope = 10/20 = 1/2 = 0.5
- The ramp's slope is '0.5'