
www.VirtualNerd.com
How Do You Write an Equation for a Vertical Line?
Write the equation of a vertical line through (2,-1)
Summary
- Now that we solved for 'b', we plug that value into the vertical line equation 'x=b'
- We want the equation of the vertical line going through the point (2,-1)
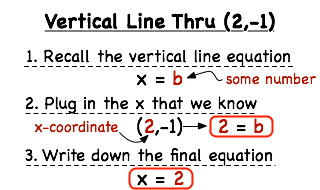
- The vertical line equation is 'x=b', where 'b' represents some number along the x-axis
- The 'x' that we know is the x-coordinate of the point (2,-1), which is '2'
- For vertical lines, we can solve for 'b' by simply plugging in our x-coordinate in for 'x'
- Now that we solved for 'b', we plug that value into the vertical line equation 'x=b'
Notes
-

-

- We want to accurately graph a vertical line through the point (2,-1)
-

- We want to accurately graph a vertical line through the point (2,-1)
-

- 'x=b' is the form of the equation that a vertical line will have
- For 'x=b', the 'b' is really what will change with each equation
- So a vertical line (straight up and down) will always intersect the x-axis, since the equation is 'x=b'
-

- 'x=b' is the form of the equation that a vertical line will have
- For 'x=b', the 'b' is really what will change with each equation
- So a vertical line (straight up and down) will always intersect the x-axis, since the equation is 'x=b'
-

- 'x=b' is the form of the equation that a vertical line will have
- For 'x=b', the 'b' is really what will change with each equation
- So a vertical line (straight up and down) will always intersect the x-axis, since the equation is 'x=b'
-

- 'x=b' is the form of the equation that a vertical line will have
- For 'x=b', the 'b' is really what will change with each equation
- So a vertical line (straight up and down) will always intersect the x-axis, since the equation is 'x=b'
- Our point (2,-1), contains the value for 'x', it's '2'
-

- 'x=b' is the form of the equation that a vertical line will have
- The '2' in (2,-1) is the x-coordinate
-

- 'x=b' is the form of the equation that a vertical line will have
- Our point (2,-1), contains the value for 'x', it's '2'
- The '2' in (2,-1) is the x-coordinate
-
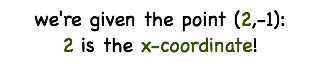
- 'x=b' is the form of the equation that a vertical line will have
- Our point (2,-1), contains the value for 'x', it's '2'
- The '2' in (2,-1) is the x-coordinate
-

- 'x=b' is the form of the equation that a vertical line will have
- Our point (2,-1), contains the value for 'x', it's '2'
- The '2' in (2,-1) is the x-coordinate
- The problem says the vertical line passes through the point (2,-1)
-

- 'x=b' is the form of the equation that a vertical line will have
- Our point (2,-1), contains the value for 'x', it's '2'
- The '2' in (2,-1) is the x-coordinate
- The problem says the vertical line passes through the point (2,-1)
-

- 'x=b' is the form of the equation that a vertical line will have
- Our point (2,-1), contains the value for 'x', it's '2'
- The '2' in (2,-1) is the x-coordinate
- The problem says the vertical line passes through the point (2,-1)
- Plugging in '2' for 'x' in the vertical line equation gives us: '2=b'
-

- 'x=b' is the form of the equation that a vertical line will have
- Our point (2,-1), contains the value for 'x', it's '2'
- The '2' in (2,-1) is the x-coordinate
- The problem says the vertical line passes through the point (2,-1)
- Plugging in '2' for 'x' in the vertical line equation gives us: '2=b'
-

- 'x=b' is the form of the equation that a vertical line will have
- Our point (2,-1), contains the value for 'x', it's '2'
- The '2' in (2,-1) is the x-coordinate
- The problem says the vertical line passes through the point (2,-1)
- Plugging in '2' for 'x' in the vertical line equation gives us: '2=b'
-

- 'x=b' is the form of the equation that a vertical line will have
- Our point (2,-1), contains the value for 'x', it's '2'
- The '2' in (2,-1) is the x-coordinate
- The problem says the vertical line passes through the point (2,-1)
- Plugging in '2' for 'x' in the vertical line equation gives us: '2=b'
-

- 'x=b' is the form of the equation the vertical line will have
-

- 'x=b' is the form of the equation the vertical line will have
- Plugging in '2' for 'x' in the vertical line equation gives us: '2=b'
- Now we plug '2' in for 'b' and get the final equation: 'x=2'
-

- 'x=b' is the form of the equation the vertical line will have
- Plugging in '2' for 'x' in the vertical line equation gives us: '2=b'
- Now we plug '2' in for 'b' and get the final equation: 'x=2'
-

- For 'x=b', the 'b' is really what will change with each equation
- Our x-coordinate was '2', so we solved for 'b' using this and were able to get the final equation: 'x=2'
-

- For 'x=b', the 'b' is really what will change with each equation
- Our x-coordinate was '2', so we solved for 'b' using this and were able to get the final equation: 'x=2'
-

- In other words, every point on the line will have an x-coordinate of '2'