
www.VirtualNerd.com
How Do You Check if a Point is on a Line If You Have a Graph?
Verify that the point (3,4) lies on the line with equation y = 2x-2
Summary
- Our first step is to graph the line of our equation 'y=2x-2' on a coordinate plane
- The y-intercept of our line is '-2', so the line must pass through the point (0,-2)
- Since the slope, 'm', is '2', we can plot a second point up 2 and to the right 1
- We can repeat this process of plotting points since the slope is constant
- Connecting the dots will give us the graphed line of our equation
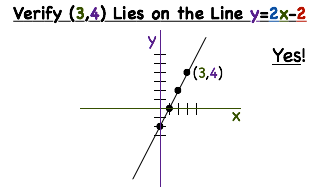
- Looking at the graphed line, we see that the point (3,4) does lie on the our line!
Notes
-

-

- The equation 'y=2x-2' is in slope-intercept form, which will make it easier to graph
- Slope-intercept form is:
- y=mx+b
-

- An x and y-coordinate axis make up what is called a coordinate plane
- We can graph our line 'y=2x-2' on this coordinate plane
-

- Slope-intercept form is:
- y=mx+b
-

- Slope-intercept form is:
- y=mx+b
- The y-intercept, 'b', is where the line of our equation passes through the y-axis
- Our equation is 'y=2x-2'
- Since '-2' is our y-intercept, we know (0,-2) falls on the line of our equation
- So (0,-2) is the first point we plot
-

- Our equation is 'y=2x-2', so the y-intercept, 'b', is '-2', and the slope, 'm', is '2'
- (0,-2) is the first point we plotted
-

- Our equation is 'y=2x-2', so the y-intercept, 'b', is '-2', and the slope, 'm', is '2'
- (0,-2) is the first point we plotted
- A slope of '2' is the same as a slope of '2 over 1', which means we can keep plotting points up 2 and right 1
-

- The dots represent the points: (0,-2), (1,0), (2,2), (3,4)
- Connecting the dots gives us the line of our equation 'y=2x-2'
-

- The dots represent the points: (0,-2), (1,0), (2,2), (3,4)
- Connecting the dots gives us the line of our equation 'y=2x-2'
-

- (3,4) means the x-coordinate will be '3' and the y-coordinate will be '4'
-

- (3,4) means the x-coordinate will be '3' and the y-coordinate will be '4'
-

- (3,4) means the x-coordinate will be '3' and the y-coordinate will be '4'
-

- (3,4) means the x-coordinate will be '3' and the y-coordinate will be '4'
- So (3,4) DOES lie on the line with the equation 'y=2x-2'
-
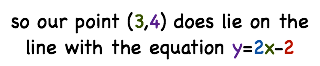
- We know this just by looking at the graph itself
- Drawing the line correctly is key to this problem!