
www.VirtualNerd.com
How Do You Check if a Point is on a Line If You Have an Equation?
Verify that the point (3,4) lies on the line with equation y=2x-2
Summary
- Writing out the equation of our line, we see that we need values for 'x' and 'y'
- We plug the x and y coordinates from our point, (3,4), in for the 'x' and 'y' in our equation
- 2(3)=6
- 6-2=4
- '4=4' is a true statement, so (3,4) DOES lie on the line with the equation 'y=2x-2'
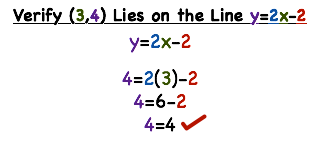
Notes
-

-

- Here, 'y=2x-2' is the equation of a line in slope-intercept form
-

- Our equation is 'y=2x-2'
- Since (3,4) is our point, we can plug it into our equation
- The x-coordinate of (3,4) is '3', and the y-coordinate is '4'
-

- Our equation is 'y=2x-2'
- Since (3,4) is our point, we can plug it into our equation
- The x-coordinate of (3,4) is '3', and the y-coordinate is '4'
- A true statement is when the values on both sides of the equal sign are the same
-

- Our equation is 'y=2x-2'
- Since (3,4) is our point, we can plug it into our equation
- The x-coordinate of (3,4) is '3', and the y-coordinate is '4'
- A true statement is when the values on both sides of the equal sign are the same
-

- Our equation is 'y=2x-2'
- Since (3,4) is our point, we can plug it into our equation
- The x-coordinate of (3,4) is '3', and the y-coordinate is '4'
-

- Our equation is 'y=2x-2'
- Since (3,4) is our point, we can plug it into our equation
- The x-coordinate of (3,4) is '3', and the y-coordinate is '4'
- After plugging in our point, 'y=2x-2' becomes '4=2(3)-2'
-

- Our equation is 'y=2x-2'
- Since (3,4) is our point, we can plug it into our equation
- The x-coordinate of (3,4) is '3', and the y-coordinate is '4'
- After plugging in our point, 'y=2x-2' becomes '4=2(3)-2'
- 2(3)=6
-

- Our equation is 'y=2x-2'
- Since (3,4) is our point, we can plug it into our equation
- The x-coordinate of (3,4) is '3', and the y-coordinate is '4'
- After plugging in our point, 'y=2x-2' becomes '4=2(3)-2'
- 2(3)=6
- 6-2=4
- '4=4' is a true statement!
-

- '4=4' is a true statement, so our point (3,4) does lie on the line!