
www.VirtualNerd.com
How Do You Put an Equation in Slope-Intercept Form Into Standard or Point-Slope Form?
Convert the following equation of a line in slope-intercept form to an equation in 1) standard form and 2) point-slope form: y=2x-2, passing through the point (3,4)
Summary
- y=2x-2 is in slope-intercept form
- (3,4) is a point on the line y=2x-2
- Ax+By=C is standard form, where A, B, and C are numbers and A is positive
- 2x-y=2 is in standard form
- y-y1=m(x-x1) is point-slope form, where m is the slope and (x1,y1) is a point on the line
- y-4=2(x-3) is in point-slope form
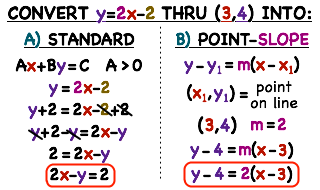
Notes
-

- Standard form of a linear equation is Ax+By=C, where A, B and C are numbers and A is positive
-

- Standard form of a linear equation is Ax+By=C, where A, B and C are numbers and A is positive
-

- Standard form of a linear equation is Ax+By=C, where A, B and C are numbers and A is positive
-

- Right now our equation is in slope-intercept form
- We want it to be in standard form
-

- Standard form of a linear equation is Ax+By=C
-

- Right now our equation is in slope-intercept form
- The general form of slope-intercept form is y=mx+b, where m is the slope and b is the y-intercept
-

- A, the number in front of the x in the standard form equation, has to be positive
- So since 2, the number we have in front of the x, is already positive, we shouldn't try to do anything to it
-

- Remember, we want to get 'x' and 'y' on one side and a number on the other side
- Since we're not moving 'x', we need to get the 2 to the other side
-

- Addition is the opposite of subtraction
- So we can add 2 to both sides to undo the subtraction
-

- We can add 2 to both sides to undo the subtraction
-

- We can add 2 to both sides to undo the subtraction
- The 2's will cancel on the right, leaving us with 2x by itself
-

- Now we have our x term by itself on one side
- But we need our x and y terms on the SAME side
-

- Remember, in standard form, x and y are on the same side of the equation
-

- We can get x and y on the same side by subtracting y from both sides
- Subtraction is the opposite of addition
-

- We can subtract y from both sides to undo the addition
- The y's will cancel on the left, leaving us with just 2
- Now we have x and y together on one side!
-

- We have x and y together on one side and a number on the other
- The equation is almost in standard form!
-

- 2=2x+y is the same as 2x+y=2
- So we can flip our equation around so it looks like the general form for standard form
-

- Now our equation is in standard form!
- Remember, standard form is Ax+By=C
- So here A=2, B=-1, and C=2
-

- Remember, standard form is Ax+By=C
-

- Point-slope form of a linear equation is y-y1=m(x-x1), where m is the slope and (x1,y1) is a point on the line
-

- Point-slope form of a linear equation is y-y1=m(x-x1), where m is the slope and (x1,y1) is a point on the line
-

- Point-slope form of a linear equation is y-y1=m(x-x1), where m is the slope and (x1,y1) is a point on the line
-

- If we have the slope and a point, all we have to do is plug them into the formula to get our equation
- m is the slope
- (x1,y1) is any point on the line
-

-

- We already know that our line goes through the point (3,4)
-

- We can plug (3,4) in for (x1,y1) in the formula for point-slope form
- So we will plug in 3 for x1 and 4 for y1
-

- All you need is a point that you know is on the line
- So if you pick a value for x and plug it into the equation of the line you have, you can find the y value that goes with that x
- Then you can use that point to plug in for (x1,y1) in point-slope form
-

- 4 is the y-coordinate of the point we were given, so we plug it in for y1
-

- 3 is the x-coordinate of the point we were given, so we plug it in for x1
- m is the slope, but we don't know that yet
-

- Our last missing piece is m, the slope
-

- Slope-intercept has the form y=mx+b, where m is the slope and b is the y-intercept
- Our original equation was y=2x-2
- So m, the slope, is 2
- And b, the y-intercept, is -2
-

- Our original equation was y=2x-2
- So m, the slope, is 2
- And b, the y-intercept, is -2
-

- We know that m is the slope, which is 2
- Since we are working with the same line, we will have the same slope no matter what form the equation is in
-

- We can use this value for the slope in our point-slope equation
-

- Remember, our slope is 2
- So we can just plug 2 in for m into our point-slope equation
-

- Plug 2 in for m
- This gives us our equation in point-slope form!
-

- Our equation in point-slope form is y-4=2(x-3)
-

- Point-slope form is pretty easy if you know the slope and a point!