
www.VirtualNerd.com
How Do You Write an Equation of a Line in Slope-Intercept Form If You Have One Point and a Parallel Line?
Find an equation of the line (in slope-intercept form) that passes through (1,10) and is parallel to the graph of 2x-y = 2
Summary
- 'L1' is a variable representing our given line
- 'L2' is a variable representing the parallel line we are trying to find
- 'y=mx+b' is the form for an equation in slope-intercept form, where 'm' is the slope and 'b' is the intercept
- 'y=2x-2' is our given equation in slope-intercept form, where 'm' and 'b' are both '2'
- Parallel lines have equal slopes, so 'L2' has a slope of 2
- 'y=2x+b' is the half-finished equation for our parallel line
- We can solve for 'b' by plugging our point (1,10) into the 'y=2x+b' equation, and we get '8=b'
- The final equation for 'L2' is: 'y=2x+8'
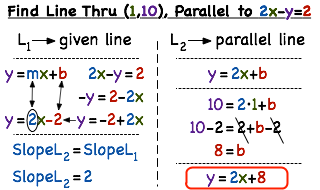
Notes
-

-

- '2x-y=2' is the line we were given, which is in standard form
-

- '2x-y=2' is the line we were given, which is in standard form
-

- Our given line can help us do this
-

- Our given line can provide us with some useful information
-

- '2x-y=2' is the line we were given, which is in standard form
-

- '2x-y=2' is the line we were given, which is in standard form
- 'y=mx+b' is the form for an equation in slope-intercept form
-

- '2x-y=2' is the line we were given, which is in standard form
- 'y=mx+b' is the form for an equation in slope-intercept form
-

- '2x-y=2' is the line we were given, which is in standard form
- 'y=mx+b' is the form for an equation in slope-intercept form
- Since we want slope-intercept form, we need to get the y alone on the left
-

- '2x-y=2' is the line we were given, which is in standard form
- 'y=mx+b' is the form for an equation in slope-intercept form
- Since we want slope-intercept form, we need to get the y alone on the left
-

- '2x-y=2' is the line we were given, which is in standard form
- 'y=mx+b' is the form for an equation in slope-intercept form
- Since we want slope-intercept form, we need to get the y alone on the left
- When we multiply by -1, all signs flip
-

- We use the commutative property to rearrange our equation so that it more closely resembles 'y=mx+b' form
-

- 'y=mx+b' is the form for an equation in slope-intercept form, where 'm' is the slope and 'b' is the intercept
- 'y=2x-2' is now our rearranged equation for the given line
-

- Our given line can provide us with some useful information about the parallel line
-

- Our given line can provide us with some useful information about the parallel line
-

- Our given line can provide us with some useful information about the parallel line
- We know the slope of our given line, it's 2!
-

-

- 'y=2x-2' is our rearranged equation for the given line
- 'y=mx+b' is the form for an equation in slope-intercept form, where 'm' is the slope and 'b' is the intercept
-

- Remember our trick says that parallel lines have the same slope!
- We know the slope of our given line, it's 2!
-

- We want our parallel line to have the 'y=mx+b' form
- We can substitute '2' in for 'm' in the 'y=mx+b' equation for our parallel line
-

- In the 'y=mx+b' equation, 'b' is the intercept
-

- We want our parallel line to have the 'y=mx+b' form
-

- We want our parallel line to have the 'y=mx+b' form
- The slope of the parallel line is the same as the given line, so it's 2!
-

- We want our parallel line to have the 'y=mx+b' form
- The slope of the parallel line is the same as the given line, so it's 2!
- So far, our parallel line looks like this: 'y=2x+b'
-

- We want our parallel line to have the 'y=mx+b' form
- The slope of the parallel line is the same as the given line, so it's 2!
- So far, our parallel line looks like this: 'y=2x+b'
-

- So far, our parallel line looks like this: 'y=2x+b'
- Here, 'b' is the y-intercept
-

- So far, our parallel line looks like this: 'y=2x+b'
- We want to figure out what the intercept ('b') is
- Since we know that the parallel line will "intercept" the point (1,10), this information will help us find 'b'
-

- For the point (1,10), the x-coordinate is '1' and the y-coordinate is '10'
- Here, 'b' is the y-intercept
-

- So far, our parallel line looks like this: 'y=2x+b'
-
Pluggin in '1' for 'x' and '10' for 'y', we get the equation: '10=2
• 1+b'
-
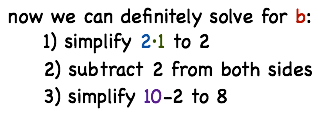
-
Pluggin in '1' for 'x' and '10' for 'y', we get the equation: '10=2
• 1+b' - Simplifying, the equation becomes: '10=2+b'
- Subtracting 2 from both sides gives us: '10-2=2-2+b'
- Simplifying again, we get: '8=b'
-
Pluggin in '1' for 'x' and '10' for 'y', we get the equation: '10=2
-

- Remember, we solved for 'b' by just plugging our point (1,10) into the half-finished equation for our parallel line
- Here, 'b' is the y-intercept
-

- Up to this point, our parallel line looked like this: 'y=2x+b'
- Now we have 'b', which is '8', for our parallel line
-

- So our full equation for the parallel line is: 'y=2x+8'