
www.VirtualNerd.com
How Do You Write an Equation of a Line in Slope-Intercept Form from a Word Problem?
Leo's tutoring company profited $1200 after one month of business and $5800 after five months. Assume that his company's profit can be modeled by a straight line. Write an equation in slope-intercept form for the graph of his company's profit over time.
Summary
- The '1' in 'x1' and 'y1' means we're talking about our 1st ordered pair
- The '2' in 'x2' and 'y2' means we're talking about our 2nd ordered pair
- (1,1200) is our 1st point, or ordered pair, on the line, and (5,5800) is our 2nd point
- Here, 'm' represents slope, which is the change in 'y' over the change in 'x'
- Slope-intercept form is: y=mx+b
- Use one of the points to solve for the y-intercept, 'b'
- The profit in slope-intercept form is: y=1150x+50
- Use the other point to check your answer

Notes
-

-

- Knowing some x and y-coordinates could help us draw a straight line
-

- Knowing some x and y-coordinates could help us draw a straight line
- A pair of x and y-coordinates, also called an ordered pair, means the same as a "point"
-

- 'x' and 'y' are variables found in the equation of a line in slope-intercept form
- So any time we're talking about time (in months), that value will be an x-coordinate on our line
- Any time a profit is mentioned, we know that value will be a y-coordinate on the line
-
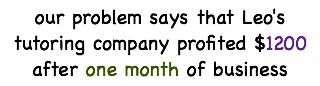
- Any time we're talking about time (in months), that value will be an x-coordinate on our line
- Any time a profit is mentioned, we know that value will be a y-coordinate on the line
-

- Our problem states that after one month, the company profited $1200
- Any time a profit is mentioned, we know that value will be a y-coordinate on the line
-

- Our problem states that after one month, the company profited $1200
- Any time we're talking about time (in months), that value will be an x-coordinate on our line
- Any time a profit is mentioned, we know that value will be a y-coordinate on the line
-

- Our problem states that after one month, the company profited $1200
- Any time a profit is mentioned, we know that value will be a y-coordinate on the line
-

- Our problem states that after one month, the company profited $1200
- Any time a profit is mentioned, we know that value will be a y-coordinate on the line
- The '1' in 'y1' just symbolizes that this is the y-coordinate for the first coordinate pair we're looking at on our line
-
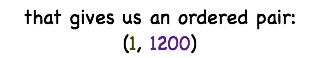
- An ordered pair is a pair of x and y-coordinates
- '1' is the x-coordinate and '1200' is the y-coordinate in this ordered pair
-

- Any time we're talking about time (in months), that value will be an x-coordinate on our line
- Any time a profit is mentioned, we know that value will be a y-coordinate on the line
-

- Our problem states that after five months, the company profited $5800
- Any time we're talking about time (in months), that value will be an x-coordinate on our line
- Any time a profit is mentioned, we know that value will be a y-coordinate on the line
-

- Our problem states that after five months, the company profited $5800
- Any time we're talking about time (in months), that value will be an x-coordinate on our line
- The '2' in 'x2' just symbolizes that this is the x-coordinate for the second coordinate pair we're looking at on our line
-

- Our problem states that after five months, the company profited $5800
- Any time a profit is mentioned, we know that value will be a y-coordinate on the line
- The '2' in 'y2' just symbolizes that this is the y-coordinate for the second coordinate pair we're looking at on our line
-

- An ordered pair is a pair of x and y-coordinates
- '5' is the x-coordinate and '5800' is the y-coordinate in this ordered pair
-

- An ordered pair is a pair of x and y-coordinates
- As long as you have at least two points, or ordered pairs, you can draw a straight line!
-

- Our two points are (1,1200) and (5,5800)
-

- Our two points are (1,1200) and (5,5800)
- So as you move along a line from one point to another, the change in value of the x-coordinate and y-coordinate is a measure of the line's slope
-

- Our two points are (1,1200) and (5,5800)
- So as you move along a line from one point to another, the change in value of the x-coordinate and y-coordinate is a measure of the line's slope
- The word 'change' here means the same as 'difference', so we can just subtract the first coordiates from the second coordinates
- y2-y1=5800-1200
- x2-x1=5-1
-

- Our two points are (1,1200) and (5,5800)
- So as you move along a line from one point to another, the change in value of the x-coordinate and y-coordinate is a measure of the line's slope
- The word 'change' here means the same as 'difference', so we can just subtract the first coordiates from the second coordinates
- y2-y1=5800-1200=4600
- x2-x1=5-1=4
- The slope is: 4600/4=1150
-

- y2-y1=5800-1200=4600
- x2-x1=5-1=4
- The slope is: 4600/4=1150
-

- The slope is: 4600/4=1150
- Our two points are (1,1200) and (5,5800)
-

- Slope-intercept form is: y=mx+b
-

- Slope-intercept form is: y=mx+b
- The 'x' and 'y' can be one of our two ordered pairs, or points
- The 'm' is the slope and the 'b' is the y-intercept
-

- Slope-intercept form is: y=mx+b
- The 'x' and 'y' can be one of our two ordered pairs, or points
- The 'm' is the slope and the 'b' is the y-intercept
- m=1150
-

- Slope-intercept form is: y=mx+b
- The 'x' and 'y' can be one of our two ordered pairs, or points
- The 'm' is the slope and the 'b' is the y-intercept
- m=1150
-

- Slope-intercept form is: y=mx+b
- The 'x' and 'y' can be one of our two ordered pairs, or points
- The 'm' is the slope and the 'b' is the y-intercept
- m=1150
- So far, our equation in slope-intercept looks like: y=1150x+b
-

- Slope-intercept form is: y=mx+b
- The 'x' and 'y' can be one of our two ordered pairs, or points
- The 'm' is the slope and the 'b' is the y-intercept
- m=1150
- So far, our equation in slope-intercept looks like: y=1150x+b
- Once we plug in values for 'x' and 'y', we will only have one unknown variable left, 'b', and that's what we need to solve for
-

- So far, our equation in slope-intercept looks like: y=1150x+b
- Once we plug in values for 'x' and 'y', we will only have one unknown variable left, 'b', and that's what we need to solve for
- Let's plug in '1' for 'x' and '1200' for 'y' in our equation
-

- So far, our equation in slope-intercept looks like: y=1150x+b
- Once we plug in values for 'x' and 'y', we will only have one unknown variable left, 'b', and that's what we need to solve for
- Plugging in '1' for 'x' and '1200' for 'y' in our equation, we get 1200=1150(1)+b
-

- Our equation looks like this: 1200=1150(1)+b
- 1150(1)=1150
- 1200-1150=50
- 50=b
-

- The y-intercept, 'b', of the company's profit line is '50'
-

- Now we're going to take out the values for 'x' and 'y' in our equation and plug in '50' for 'b'
- The y-intercept, 'b', of the company's profit line is '50'
-

- We took out the values for 'x' and 'y' in our equation and plugged in '50' for 'b'
- The y-intercept, 'b', of the company's profit line is '50'
- '1150' is our slope, 'm'
- Slope-intercept form is 'y=mx+b', so we now have an equation for our line in slope-intercept form
- Now all you need is either an x-coordinate or a y-coordinate and you can find the other coordinate for that point on the line
-

- The equation for the company's profit is: y=1150x+50
- Slope-intercept form is 'y=mx+b', so we now have an equation for our line in slope-intercept form
- Now all you need is either an x-coordinate or a y-coordinate and you can find the other coordinate for that point on the line
-

- The equation for the company's profit is: y=1150x+50
- We found this equation using one of our two ordered pairs
- Since the other ordered pair falls on the same line, we can plug its x and y-coordinates into our new equation to see if we get a true statement
- A true statement will tell us that our equation is correct
-

- The equation for the company's profit is: y=1150x+50
- We found this equation using one of our two ordered pairs
- Since the other ordered pair falls on the same line, we can plug its x and y-coordinates into our new equation to see if we get a true statement
- A true statement will tell us that our equation is correct
-

- The equation for the company's profit is: y=1150x+50
- We found this equation using one of our two ordered pairs
- Since the other ordered pair falls on the same line, we can plug its x and y-coordinates into our new equation to see if we get a true statement
- A true statement will tell us that our equation is correct
-

- Our two points are (1,1200) and (5,5800)
- Since we found our equation using (1,1200), we can check to make sure it's correct by using (5,5800) since the two points are on the same line
- x=5
- y=5800
- 1150(5)=5750
- 5750+50=5800
-

- '5800=5800' is a true statement
- A true statement tells that our equation is correct
-

- We found this equation using one of our two ordered pairs
- Now all you need is either an x-coordinate or a y-coordinate and you can find the other coordinate for that point on the line