
www.VirtualNerd.com
How Do You Divide Two Polynomials by Factoring and Canceling?
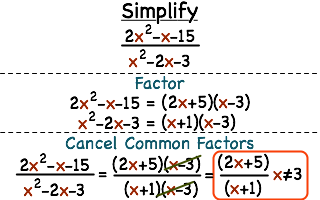
Simplify the rational expression 2x2-x-15 divided by x2-2x-3.
Summary
- Factoring the numerator and denominator will allow us to find and cancel out common factors
- The numerator is 2x2-x-15
- The denominator is x2-2x-3
- Rewrite the numerator and denominator in factored form to cancel out common factors
- If x=3 the denominator would be zero, so x cannot equal 3
- 3 is called an 'excluded value'
Notes
-

- The numerator is 2x2-x-15
- The only way to get 2x2 using two linear terms is to multiply 2x by x!
- +5 and -3 work because +5•(-3) = -15, and 5•x-3•2x=-x!
-

- We can FOIL our two binomials together to see if we get the original trinomial back
- Also check out the last link below to see a cool alternative to the FOIL method!
-

- FOIL (2x+5) and (x-3) together to get the numerator 2x2-x-15 back
- Multiply the first terms, 2x•x, to get 2x2
- Multiply the outer terms, 2x•-3, to get -6x
- Multiply the inner terms, 5•x, to get 5x
- Multiply the last terms, 5•-3, to get -15
- Simplifying gives us our original numerator back, so we factored correctly!
-

- The denominator is x2-2x-3
-

- The denominator is x2-2x-3
- We need to find a pair of numbers that multiply to -3 and add to -2
- The factors -3 and 1 give us exactly what we need in the original polynomial
- So we can use those factors to complete the binomals
-

- We factored the numerator into (2x+5)(x-3)
- We factored the denominator into (x+1)(x-3)
-

- Right now we have (2x+5)(x-3) being divided by (x+1)(x-3)
- The two (x-3)'s can cancel out to give us our answer!
-

- We can't let the denominator be zero because we're not allowed to divide by zero
- So we need to exclude 3 from our solution
- We call 3 an 'excluded value'