
www.VirtualNerd.com
How Do You Divide Quotients of Monomials?
Divide these rational expressions:
Summary
- Change the division to multiplication using the reciprocal rule of division
- Cancel out any common factors that you can
- Rewrite the factors that are left before you multiply
- Finally, multiply the monomials to find our answer
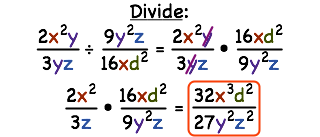
Notes
-

-
- You can always change any kind of division into multiplication by using the reciprocal rule
-

- This is the principle of the reciprocal rule of division
-

- You can only do this because of the reciprocal rule of division
-

- We need to find and then cancel out common factors in the numerator and denominator
-

- 2 is a factor in the monomial 2x2y
-

- x2 is a factor in the monomial 2x2y
-

- y is a factor in the monomial 2x2y
- y is also a factor of the monomial 3yz in the denominator
- Since y is a factor in both the numerator and denominator, we can cancel it
-

- 16 is a factor in 16xd2
-

- We already know that there are no x's in the denominator for an x to cancel with
- The x in 16xd2 just stays there.
- There is only one monomial with a d in it, so we can't cancel it out with anything else
-

- It'll be easier to multiply everything together if we know what terms are left to multiply
-

- Remember, we canceled out the y's in the original fraction
-

-

- Now we can multiply the monomials together in the numerators and denominators
-

- Multiply the monomials together in the numerators and denominators
- The numerators: 2x2•16xd2=32x3d2
- The denominators: 3z•9y2z=27y2z2
-

- Use the reciprocal rule of division to change division to multiplication
- Find factors that are in both the numerator and denominator and cancel them
- Rewriting the problem will help us multiply things later
- Multiply the numerators and denominators straight across to simplify