
www.VirtualNerd.com
How Do You Multiply Quotients of Monomials by Canceling Factors?
Simplify this expression by canceling common factors:
[(2x2y)/(3yz)]•[(9y2z)/(16xd2)]
Summary
- Cancel out any common factors that you can before multiplying
- x2 is the same as x•x, so one of its x's cancels with the x in the denominator
- 9 is the same as 3•3, so one of its 3's cancels with the 3 in the denominator
- We can rewrite the monomials in the numerator and denominator based on what factors we've canceled out
- Everything in in the first monomial in the denominator canceled, so we can just write it as 1
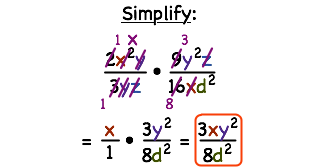
Notes
-

-

- Dividing our fractions can allow us to multiply two simpler fractions than what we started with
-

- Factors of a monomial are all the variables or prime numbers that make up the monomial
- For example, the factors of 2x2y are 2, x2 and y
-

- To avoid problems, go through one by one and find the numbers and variables that cancel
-

- 2 is the first factor of 2x2y
- 16 is the first factor of 16xd2 on the bottom
- The 2 completely cancels out, and the 16 is reduced to 8 in the denominator
-

- x2 is a factor of 2x2y
-

- x2 is a factor of 2x2y
- x is the second factor in 16xd2
- x2 is just x•x, and one of those x's can cancel with the x in the denominator
-

- y is a factor of 2x2y
- There is a y in 2x2y and 3yz, so the two y's can cancel out completely
-

- 9 is a factor of 9y2z
- One of the 3's in 9 cancels with the 3 in 3yz
-

- y2 is a factor of 9y2z
- We can't use the y in 3yz to cancel with a y in y2 since we've already used the y in 3yz
- This is partly why we suggest going slowly, you don't want to reuse variables
-

- z is a factor of both 9y2z and 3yz, so it cancels out completely!
-

- We've checked all of the factors in the numerator with all the factors in the denominator
-

- Let's make sure we know what we've canceled by rewriting what is left over
-

- We canceled out a 2, x, y, 3, and z from the numerator
- That leaves us with an x, 3, y2 on the top
- We canceled out a 3, y, z, 2, and x from the denominator
- That leaves us with 8 and d2 on the bottom
-

- The first monomial in the numerator was reduced to just the x
-

- The first monomial in the denominator canceled completely, which is why we're putting a 1 there
-

- The second monomial in the numerator reduced to 3y2
-

- The second monomial in the denominator reduced to 8d2
-

- We can now multiply the two monomials in the numerator, and the monomial in the denominator
-

- Just multiply everything straight across and combine it into one term
-

- To avoid problems, go through one by one and find the numbers and variables that cancel
- After you cancel, rewrite the left over factors so that you can easily multiply them together