
www.VirtualNerd.com
How Do You Use the Formula for the Product of a Sum and a Difference?
Expand the following: (2x + 3)(2x - 3)
Summary
- These two binomials are exactly the same except for their signs
- The formula for the product of a sum and difference gives us a shortcut so we don't have to FOIL
- Both 4x2 and 9 are perfect squares, which means we have a difference of squares
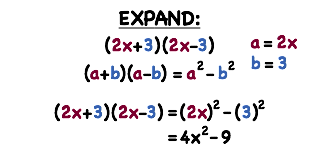
Notes
-

- The formula for the product of a sum and difference is (a+b)(a-b)=a2-b2
-

- 'a' is the first term in each binomial, which is 2x
- 'b' is the second term in each binomial, which is 3
-

- Plug '2x' in for 'a' and '3' in for 'b' into the formula for the product of a sum and difference
- We get (2x+3)(2x-3)=(2x)2-(3)2
-

- Square 2x to get 4x2
- Square 3 to get 9
-

- Since both 4x2 and 9 are perfect squares, we have a difference of squares
- You can use this formula in reverse as a shortcut to factoring a difference of squares
- Just take the square root of each of the terms and plug them in for 'a' and 'b' in the left hand side of the formula